In the realm of finance, options trading stands as a sophisticated strategy fraught with risk and reward. Navigating this intricate landscape requires a deft understanding of the underlying equations that orchestrate the ebb and flow of option pricing. Enter this comprehensive guide, where we delve into the heart of these equations, equipping you with the knowledge to venture forth confidently in the options market.
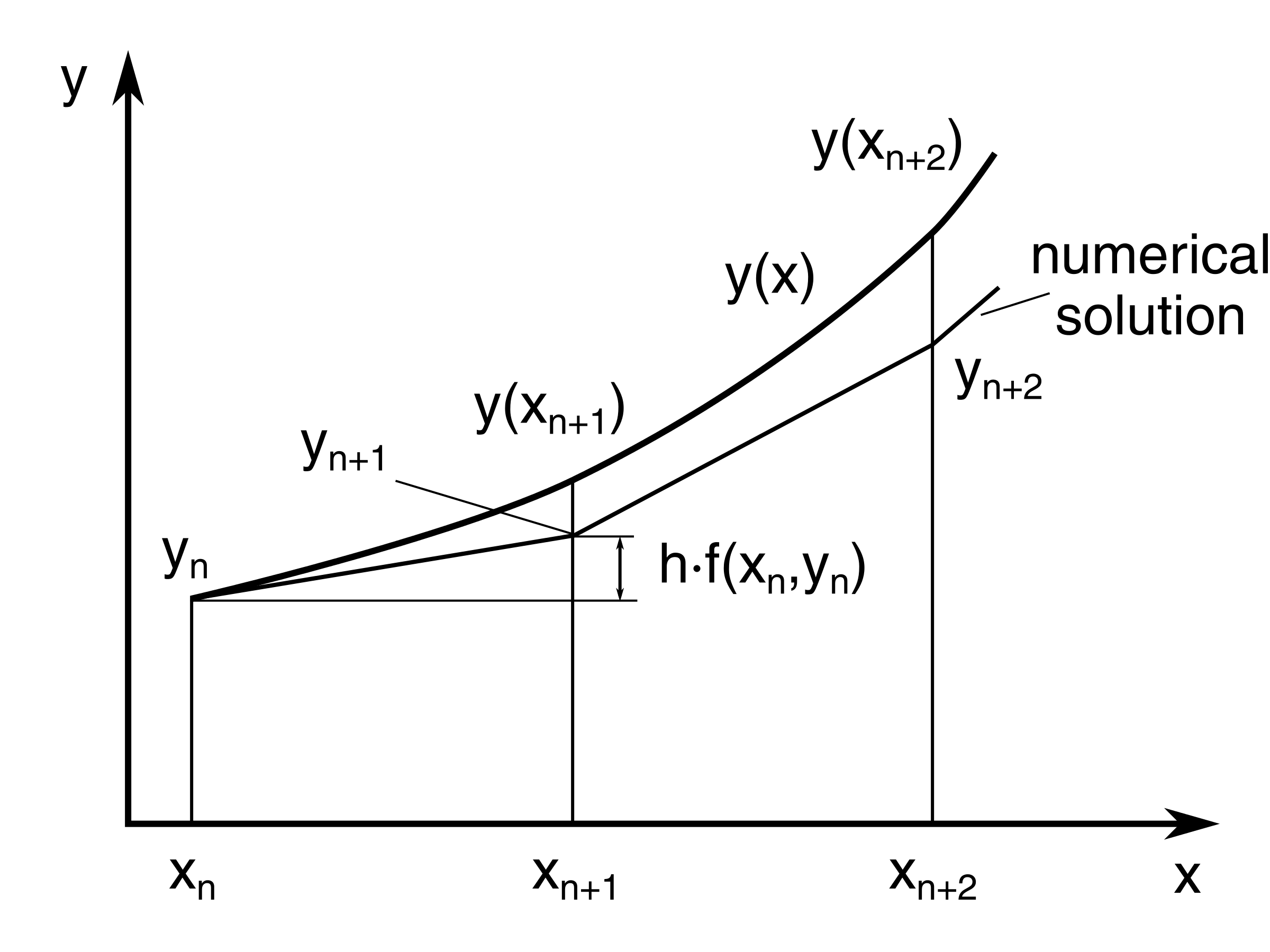
Image: guide.freecodecamp.org
Before embarking on this mathematical odyssey, let us cast our gaze upon the origins of options trading. Options emerged from the agricultural industry, where farmers sought to mitigate the vagaries of weather and harvest yields. Over time, this hedging mechanism evolved into a versatile financial instrument leveraged by investors worldwide.
The Black-Scholes Model: A Gateway to Options Pricing
Among the array of equations that govern options trading, the Black-Scholes model reigns supreme. This groundbreaking formula, concocted by Fischer Black and Myron Scholes, calculates the theoretical value of an option based on a myriad of factors, including the underlying asset’s price, volatility, time to expiration, and risk-free interest rate. The Black-Scholes model serves as the cornerstone of modern options trading, empowering investors to quantify risk and potential returns.
The Black-Scholes equation, expressed in its mathematical splendor:
C = S * N(d1) - K * e^(-rT) * N(d2)Where:
- C represents the theoretical value of the call option
- S symbolizes the current stock price
- K denotes the strike price of the option
- T represents the time remaining until the option expires
- r signifies the risk-free interest rate
- N(x) represents the cumulative distribution function of the standard normal distribution
- d1 = [ln(S/K) + (r + σ^2/2) T] / (σ √T)
- d2 = d1 – σ * √T
The Greeks: Unraveling the Dynamics of Options
While the Black-Scholes model serves as a foundational framework, a deeper understanding of options trading necessitates an acquaintance with the Greeks. These metrics measure the sensitivity of an option’s price to changes in various underlying factors, providing invaluable insights into risk and reward.
Meet the Greek pantheon:
- Delta (Δ): Measures the change in an option’s price for a one-unit change in the underlying asset’s price.
- Gamma (Γ): Captures the change in Delta for a one-unit change in the underlying asset’s price.
- Vega (ν): Quantifies the change in an option’s price for a 1% change in volatility.
- Theta (θ): Measures the decay in an option’s value as time passes.
- Rho (ρ): Reflects the change in an option’s price for a 1% change in the risk-free interest rate.
Tips for Mastering Options Trading Equations
Embarking on the journey of options trading demands a calculated approach. Harness these expert tips to navigate the complexities of options equations:
- Comprehend the Black-Scholes model: Grasping the essence of the Black-Scholes equation is non-negotiable for successful options trading.
- Scrutinize the Greeks: Familiarize yourself with the Greeks and their implications. This knowledge empowers you to assess risk and make informed trading decisions.
- Practice diligently: The path to mastery requires consistent practice. Utilize online calculators or spreadsheets to hone your skills in applying options pricing equations.
- Leverage trading simulators: Immerse yourself in risk-free trading environments, such as trading simulators, to test your strategies and refine your understanding of options equations.

Image: www.sellercourse.com
Frequently Asked Questions
To illuminate the intricacies of options trading equations further, we present a comprehensive FAQ encompassing commonly encountered queries:
Q: How do I calculate the implied volatility of an option?
A: Implied volatility cannot be directly computed but can be approximated using algorithms or iterative methods like the Newton-Raphson method.
Q: What strategies can I employ to manage risk in options trading?
A: Consider strategies like hedging, diversification, and adjusting position size to mitigate risk while pursuing profit.
Q: How do I choose the right strike price and expiration date for an option?
A: Determine the strike price based on your market outlook and profit objectives. Select an expiration date that aligns with your investment horizon and risk tolerance.
Equations For Trading Options
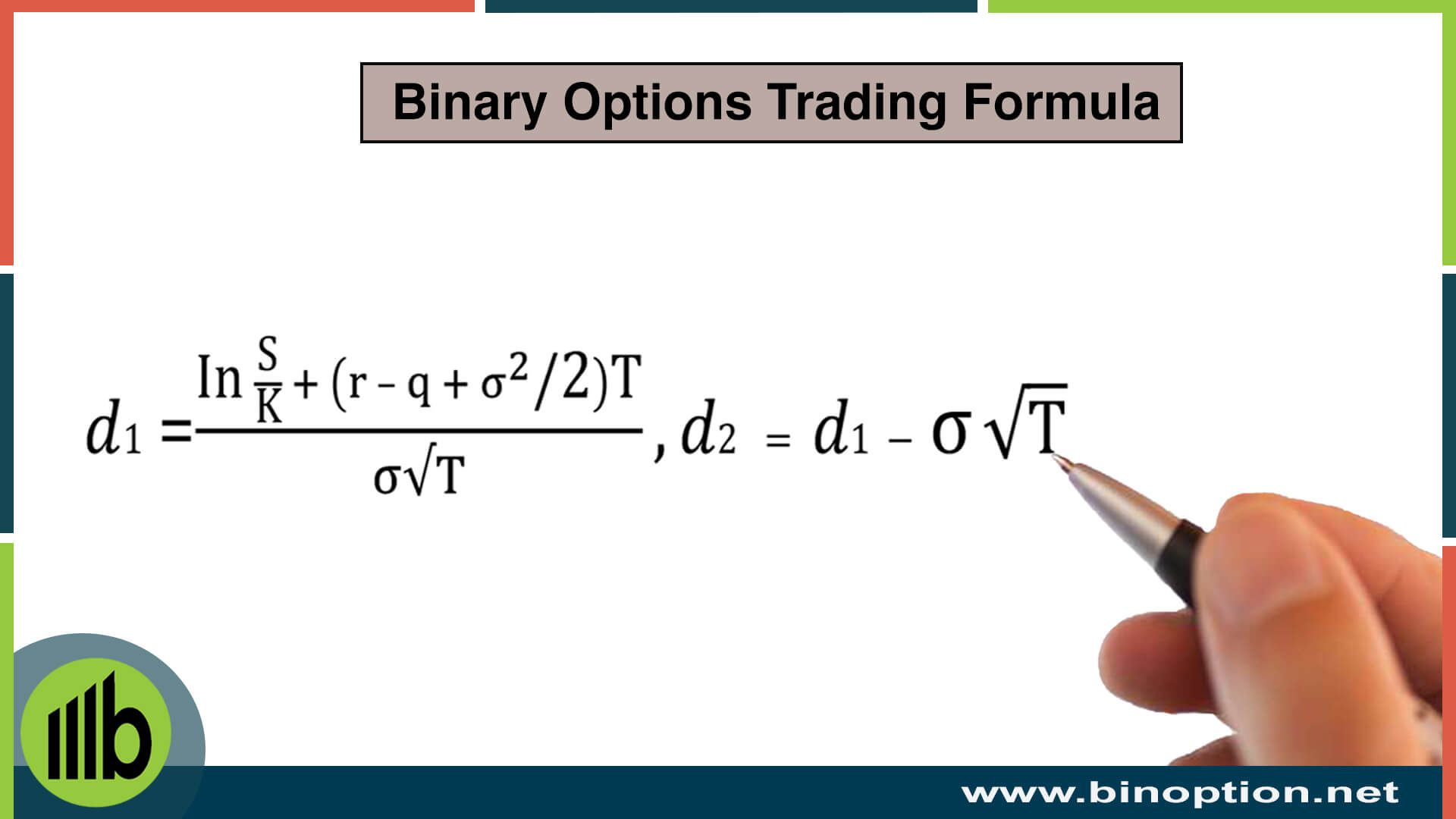
Image: www.binoption.net
Conclusion
The world of options trading presents a wealth of opportunities for discerning investors. By unlocking the power of the equations that govern this intricate market, you gain the knowledge and confidence to navigate its complexities. Whether you seek to enhance your trading acumen or simply expand your financial literacy, this comprehensive exploration into options trading equations empowers you to make informed decisions and pursue your investment goals.
Are the equations of options trading sparking your curiosity or fueling your passion for finance? Delve deeper into this fascinating realm and uncover the hidden secrets that unlock the mysteries of the market.






